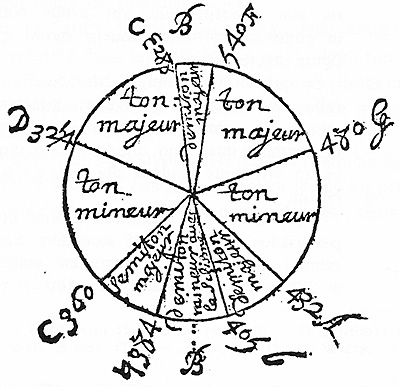
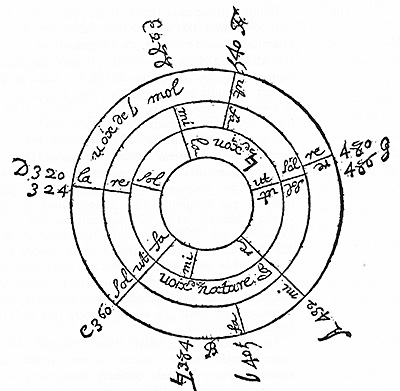
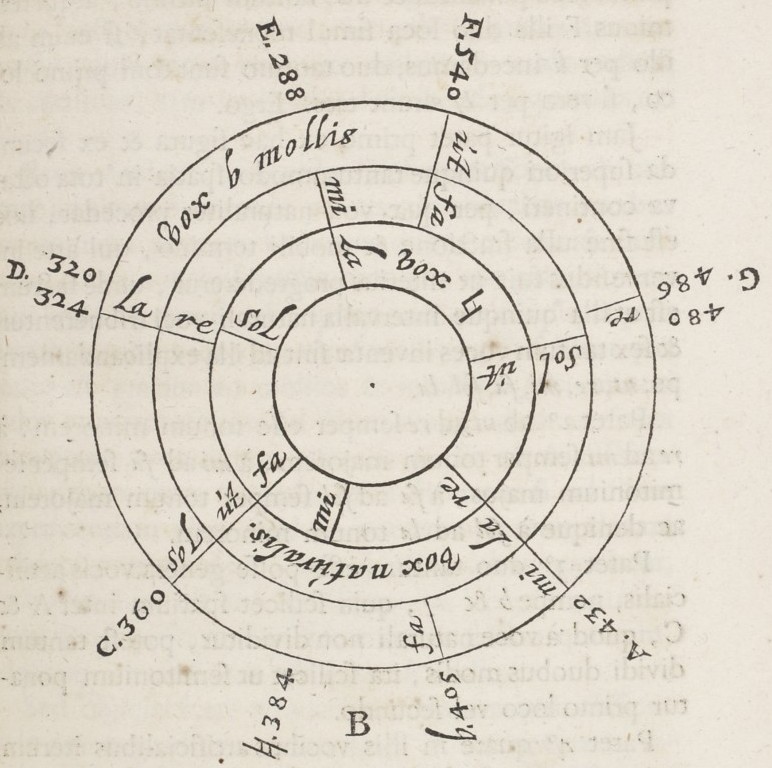
Johannes Cotto c. 1200
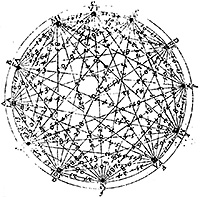
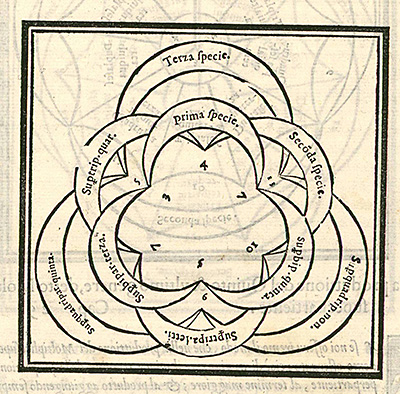
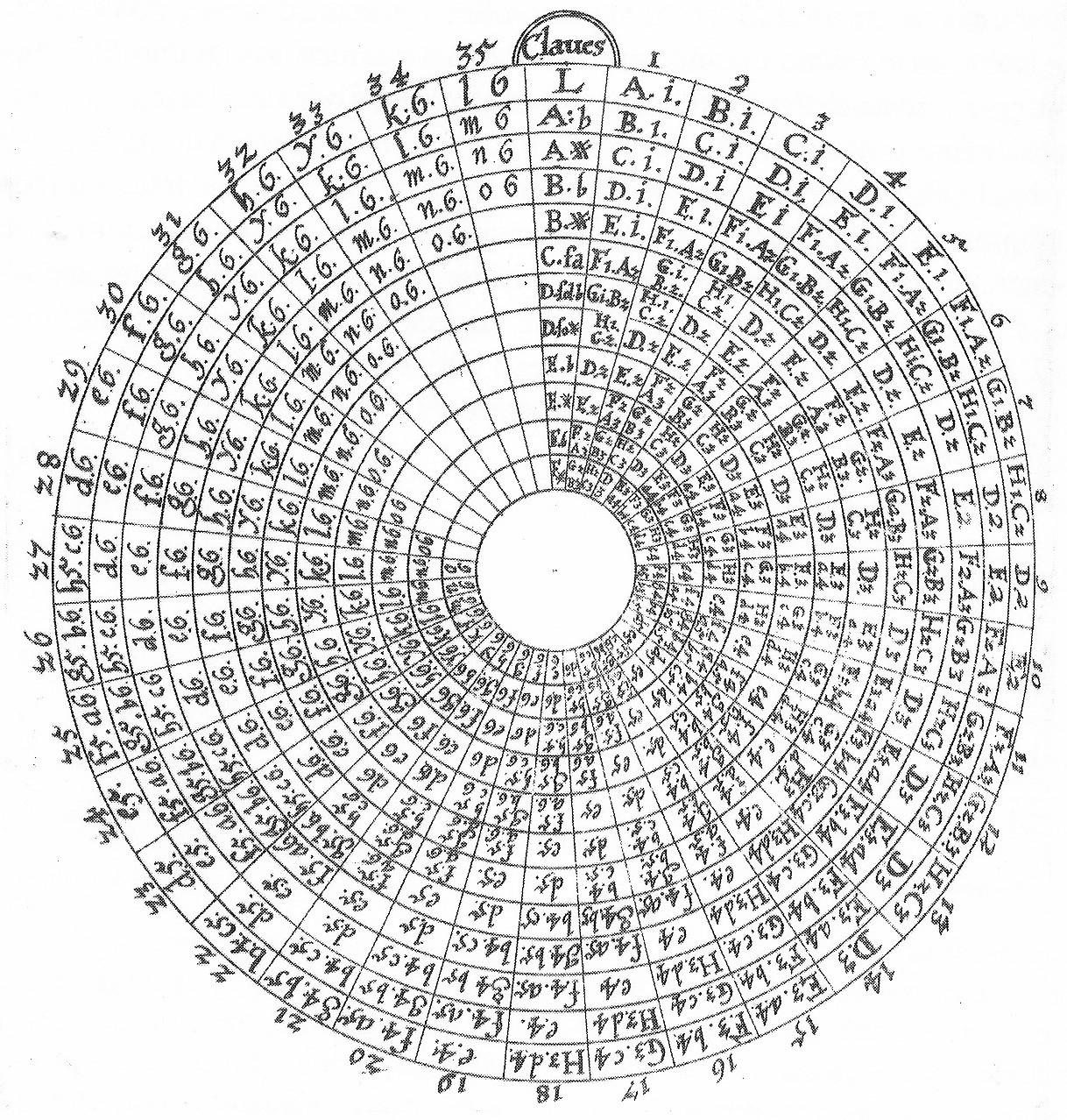
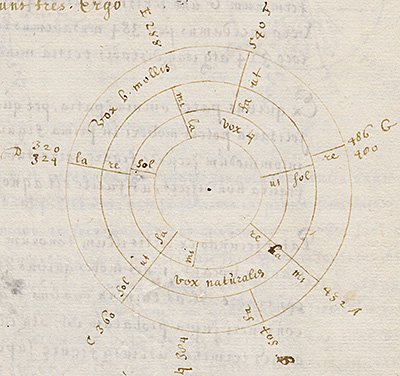
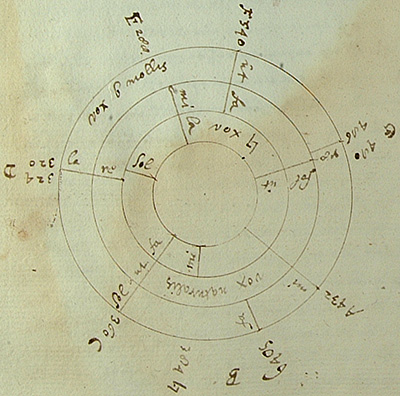
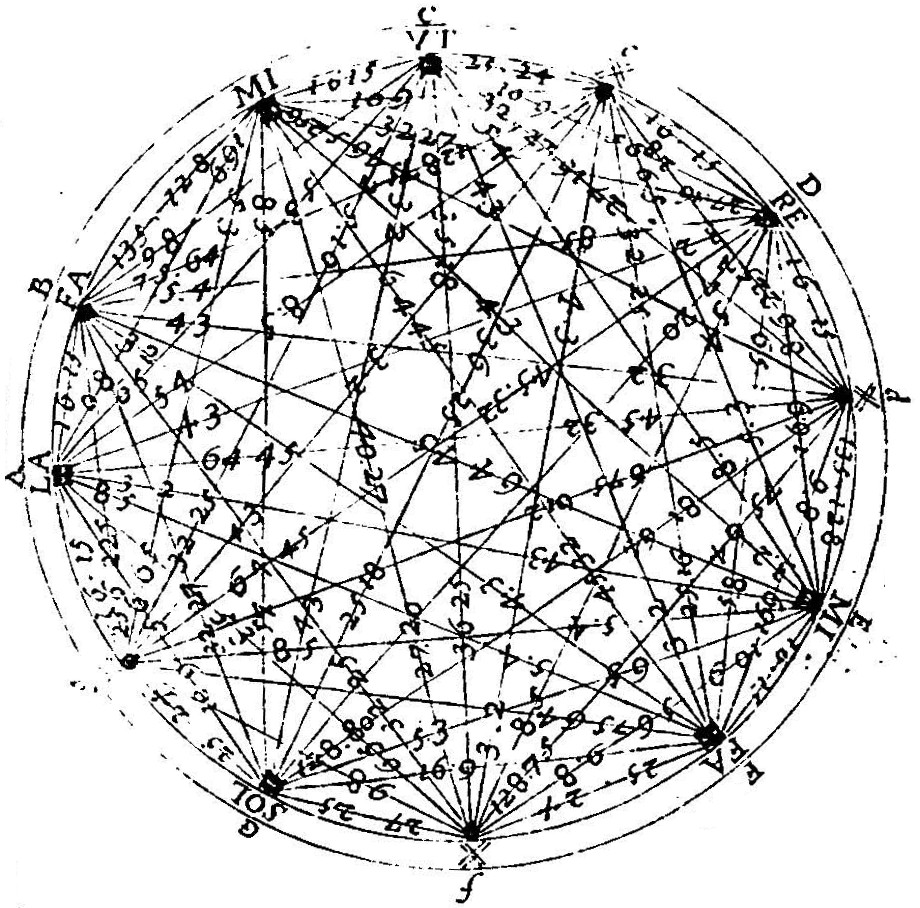
The ambits of the eight medieval modes arranged on a circle. The authentic modes are on the right, the plagal modes on the left, so that the modes with the same finalis are opposite: I / II: D (dorian / hypodorian) III / IIII: E (phrygian / hypophrygian) V / VI: F (lydian / hypolydian) VII / VIII: G (mixolydian / hypomixolydian) Whereas the modes I, V and VII include the note below the finalis, the lowest note in mode IV is the finalis F, and not E.
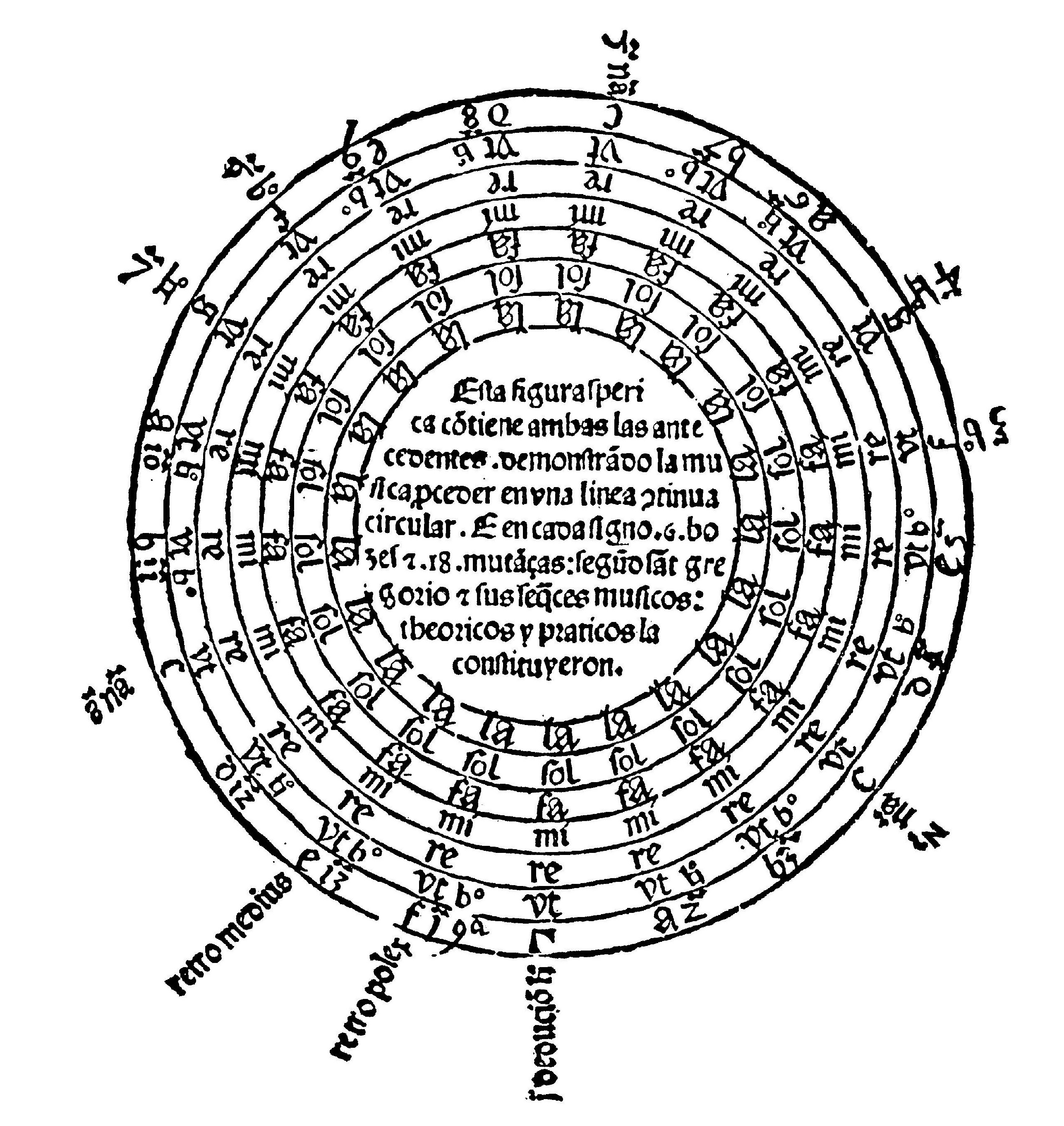
Domingo Marcos Durán 1492
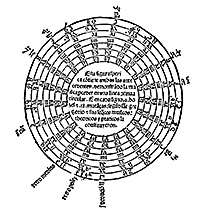
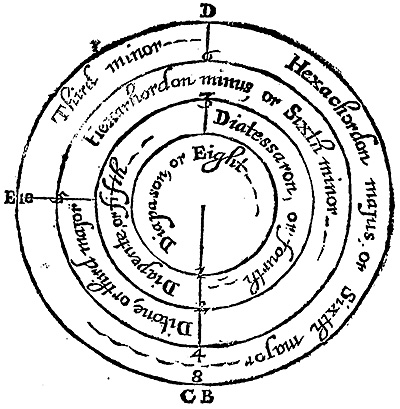
Extension of Guido of Arezzo's hexachord system. Three octaves of a diatonic scale, G-A-Bb-C-D-Eb-F, are arranged anticlockwise around a circle. From each of these pitches a hexachord ut-re-mi-fa-sol-la (with a semitone between mi and fa) is built. This procedure creates a set of twelve pitch classes C, C#, D, Eb, E, F, F#, G, Ab, A, Bb, B defining the accidentals of the musica ficta. This seems to be the earliest diagram using the circle line to represent a pitch range of three octaves. A circular diagram with three octaves appears in Galilei (1588) and a very similar diagram in Fludd (1618/24).
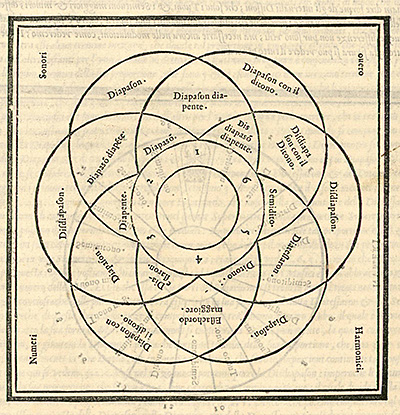
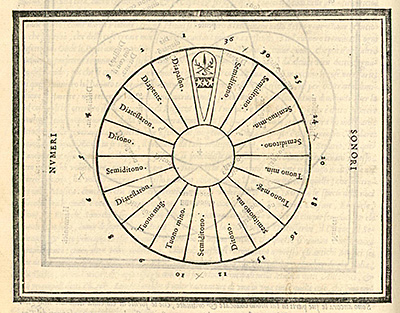
Gioseffo Zarlino 1562
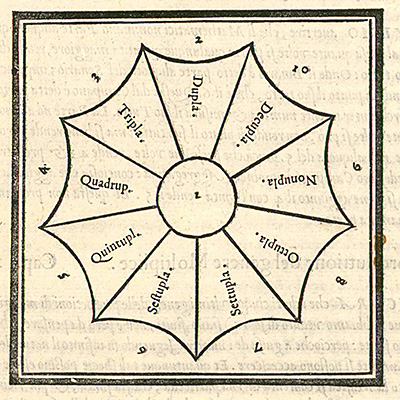
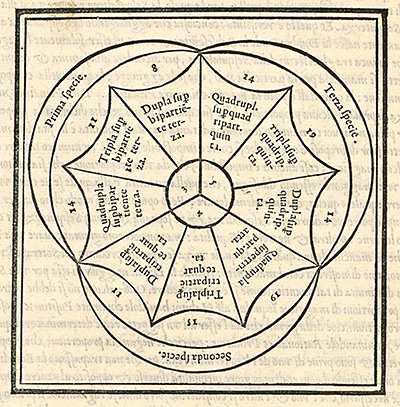
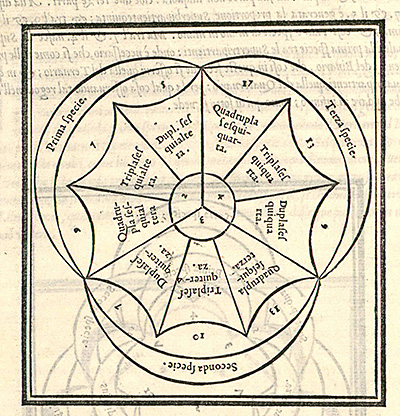
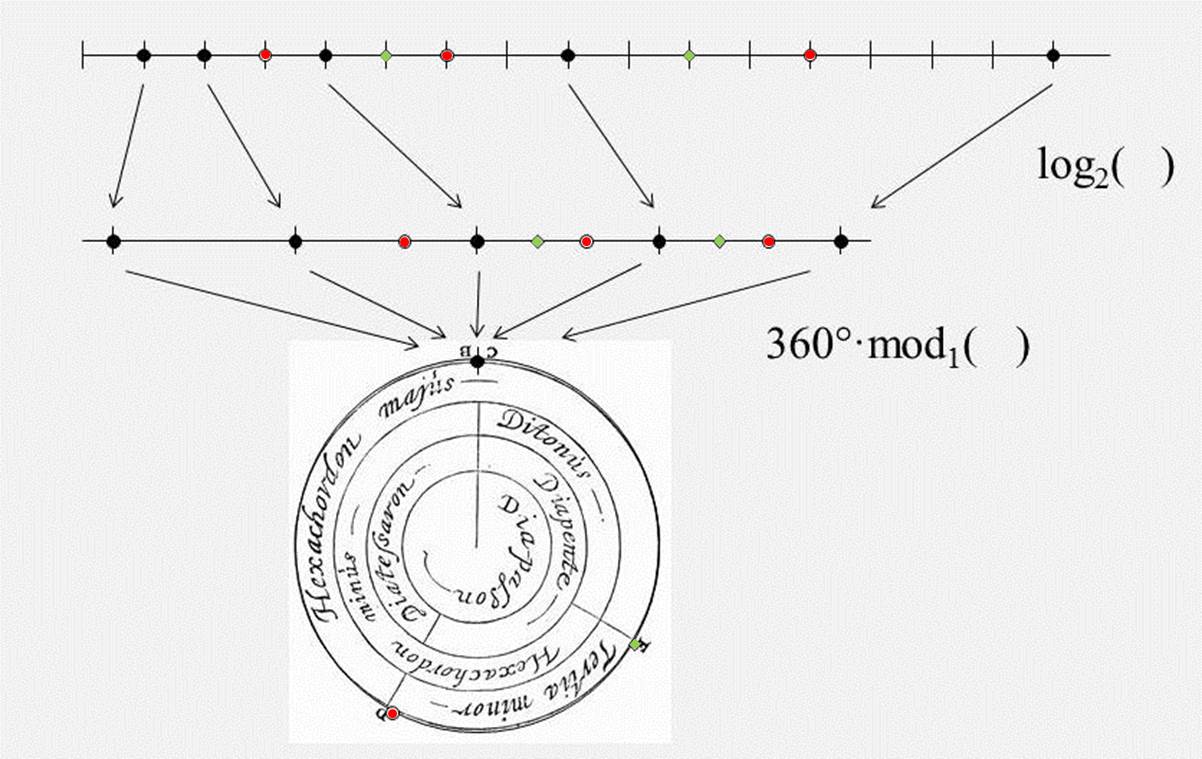
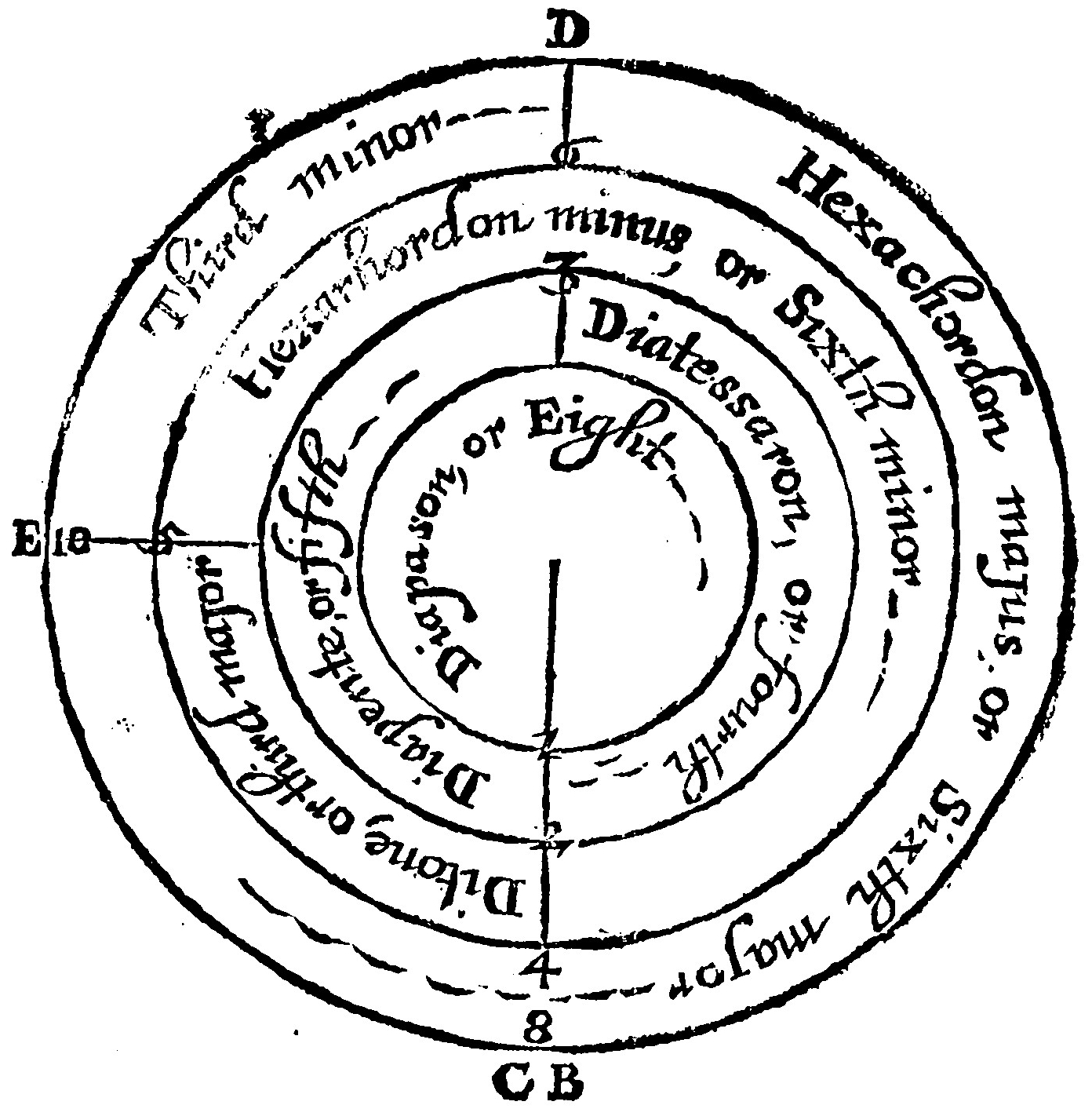
Zarlino's "senario". The "Numeri Sonori overo Harmonici" 1, 2, 3, 4, 5, 6 are arranged on a circle. Each pair of opposite numbers is connected by two big circular arcs. Their cross sections contain the names of the corresponding intervals. The ratio 6:4 should be labelled Diapente instead of Diatessaron. The senario is an extension of the Greek tetraktys (1,2,3,4), where each pair of numbers defines a consonant interval. The problem with the senario is that it does not classify the minor sixth 8:5 as a consonant interval. The ottonario 1, 2, …, 8 however would also include the number 7 to the system of consonances.
Closing the system at 6 is arbitrary, but applying a circular arrangement in order to visualize the relationships makes sense, because the system of consonances is defined by all pairs of numbers.
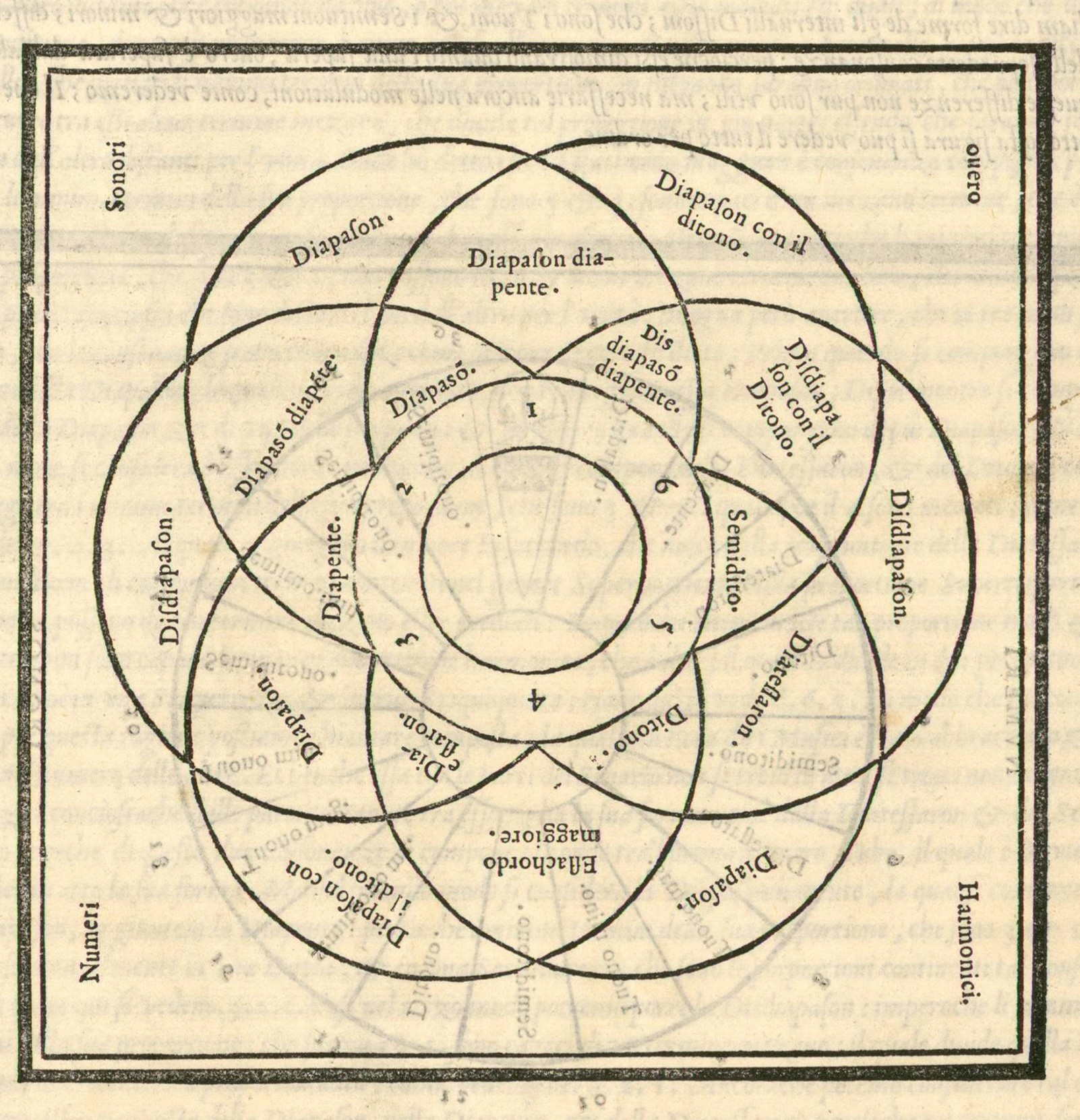
Gioseffo Zarlino 1562
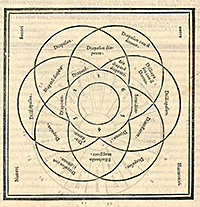
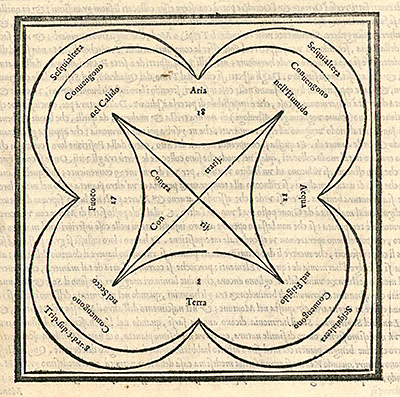
The sequence of fifths 8:12:18:27 is equated with the four elements Terra, Acqua, Aria, Fuoco. By selecting a circular arrangement the ratio 8:27 is graphically put on the same level as 3:2. The ratios between neighboured elements are also associated with the qualities Frigido, Humido, Calido, Secco. Similar diagrams could be devised with different numbers of elements and based on different ratios.
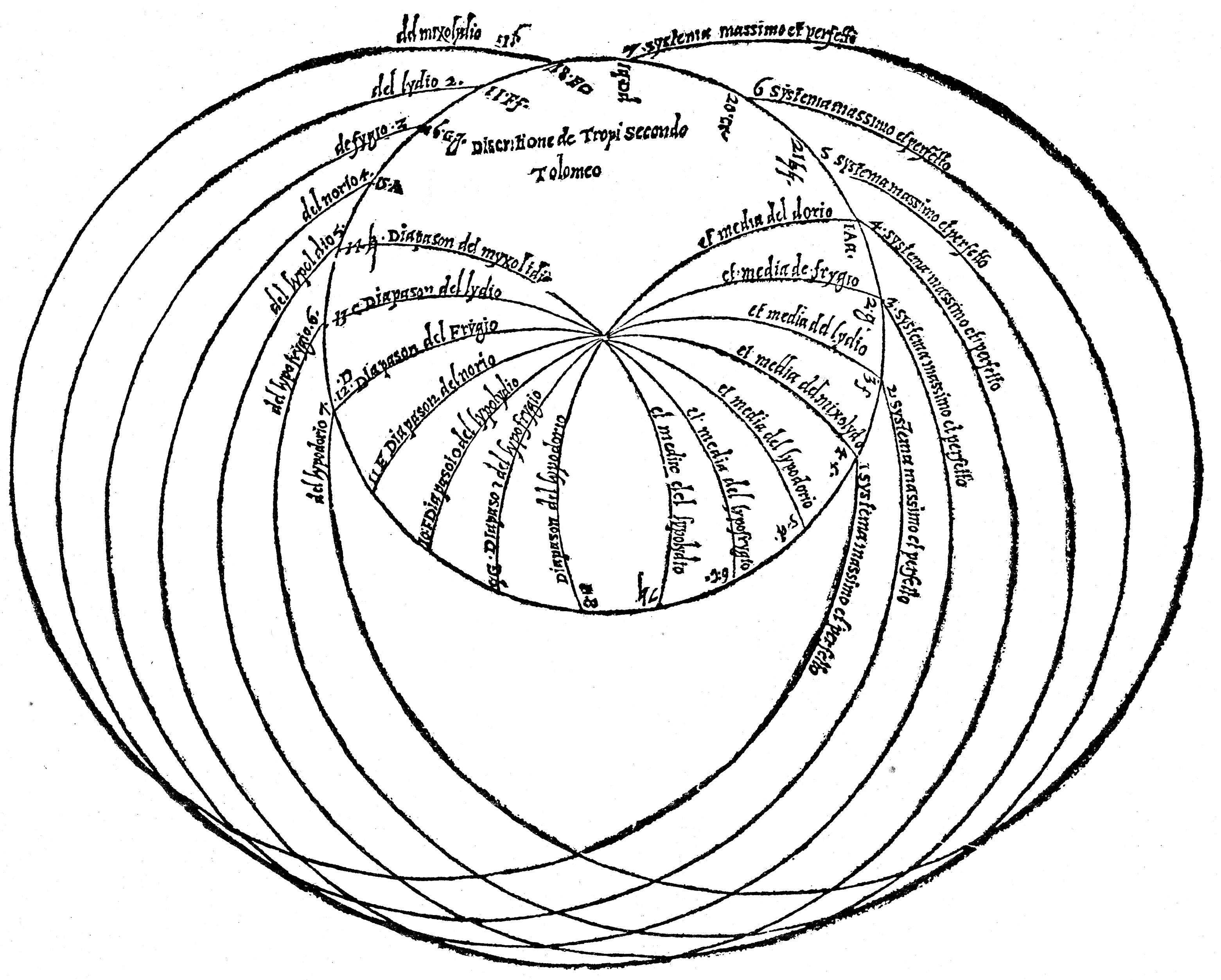
Vincenzo Galilei 1581
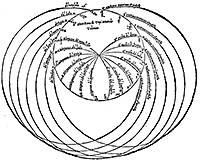
Circular arrangement of the octave species according to Ptolemy. The full circle comprises three octaves of a diatonic scale. Pitch increases anticlockwise. The pitches are numbered clockwise from 1 to 21 beginning with A ("4. systema massimo et perfetto"). The inner small circular arcs connect pitches one octave apart, the outer large arcs connect pitches two octaves apart. Because 7 is an odd number, the diagram cannot be perfectly symmetrical.
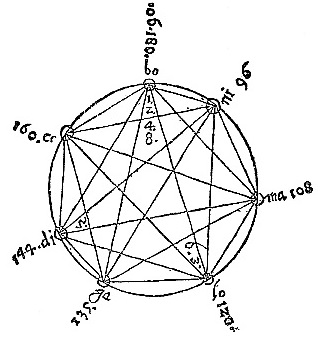
Johannes Lippius 1612
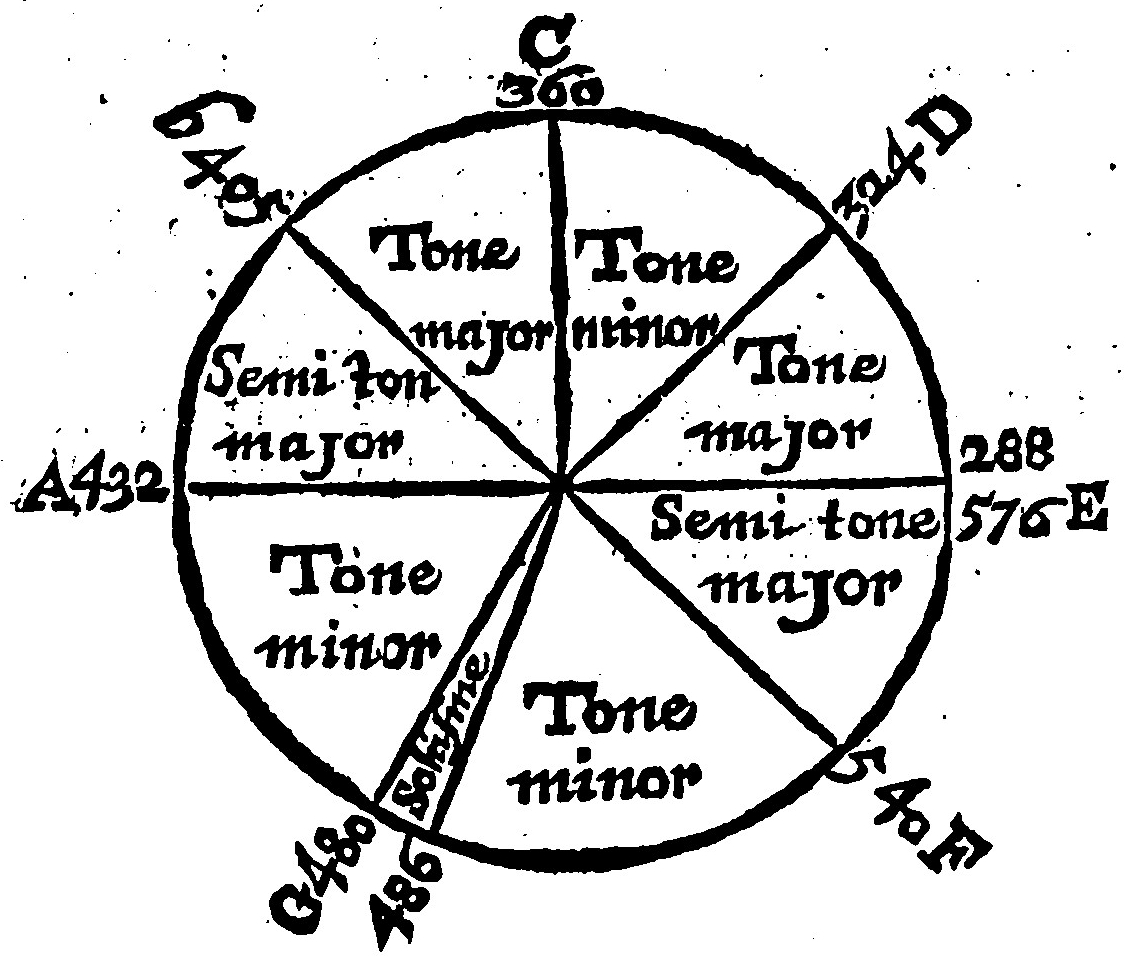
Syntonic diatonic scale on a pitch circle. This seems to be the first representation of this kind. The numbers around the circle indicate string lengths. The scale is ascending in anti-clockwise direction. Possibly Descartes (1618) and probably Mersenne (1636) were inspired by this diagram. Mersenne also connects all pairs of notes and he is familiar with the solmisation bo-ce-di-ga-lo-ma-ni-bo. This solmisation method was devised by Hubert Waelrant (about 1575).
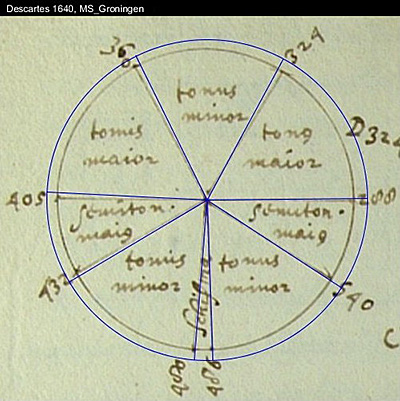
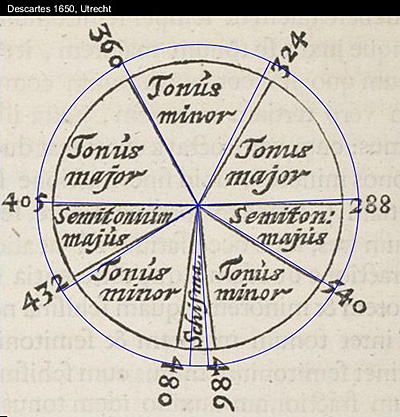
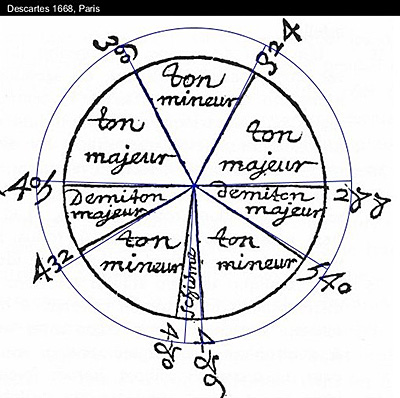
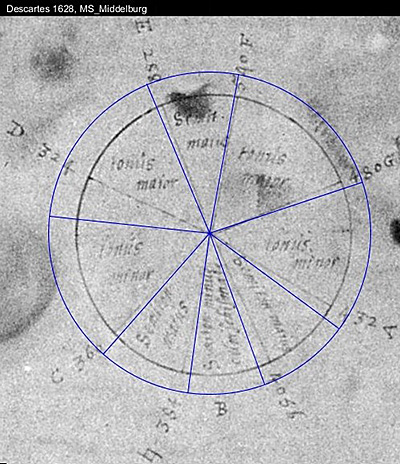
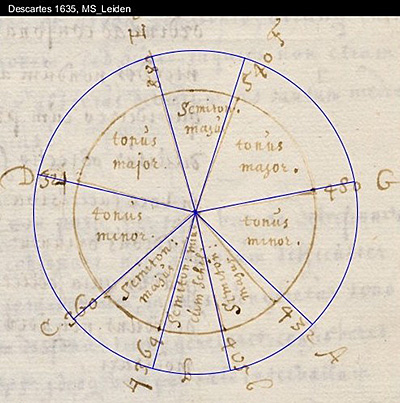
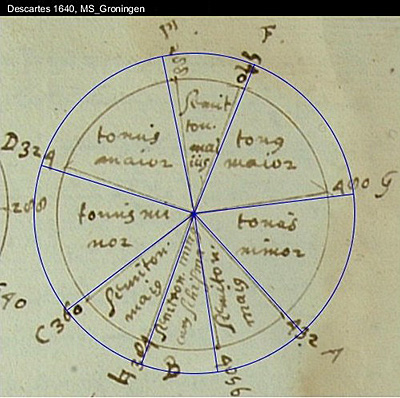
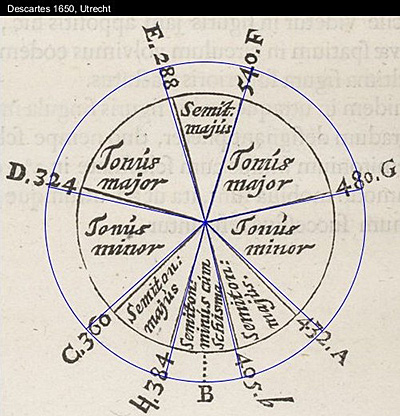
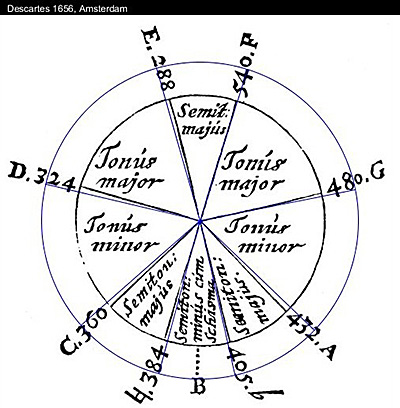
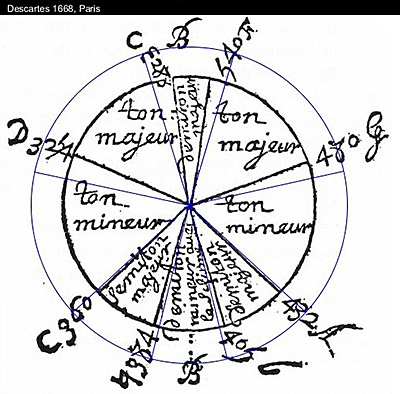
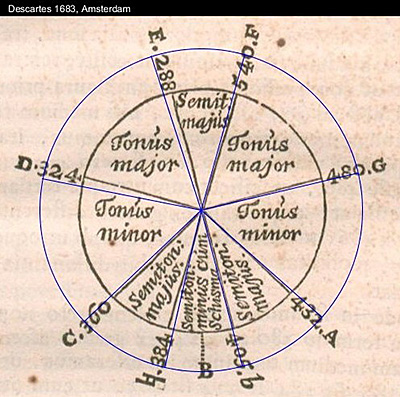
Descartes 1650
Hexachords. The final circular diagram of musical pitch from Descartes’s Compendium musicae. Three hexachords with the starting notes F, C and G are shown together. The use of just intonation means that their versions of the pitches D and G differ from one another by a syntonic comma (81:80). The three hexachords ut-re-mi-fa-sol-la have the same symmetric interval structure t-T-S-T-t *). Together they cover two major scales, on F and C (with ambiguous pitches D and G). The configuration of the 10 pitches is mirror symmetric about the vertical diameter through the middle of Bb-B and the middle of E-F. *) T = major tone (9:8); t = minor tone (10:9); S = diatonic semitone (16:15)
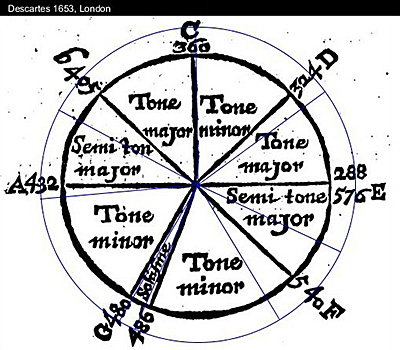
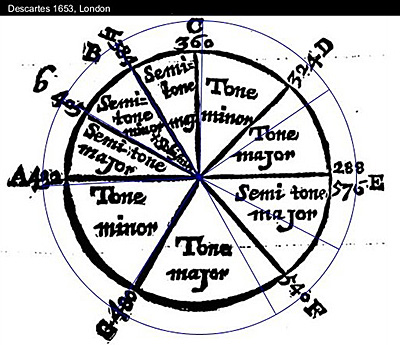
Descartes 1653
Pitch is not represented logarithmically in the English edition of Descartes’ Compendium musicae, so that equal musical intervals do not correspond to equal angles.
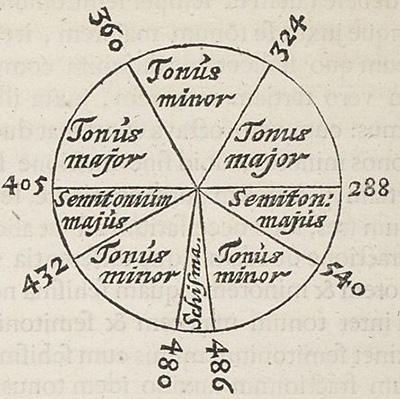
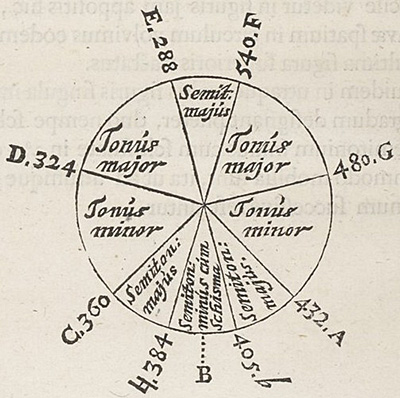
Both the fifth and the fourth measure 180° and both thirds, the major and the minor, measure 90°. The circle defined by the numbers 2, 3 (, 4) represents half a monochord string of length 4. Likewise, 4, 5, 6 (,8) indicate half a monochord string of length 8.
The circumference corresponds to one halve of a string of length 576. The string is attached at E = 576, its middle is at E = 288, the position of the bridge corresponds to 0 (not shown). The angles of the semitone E-F and the major tone D-E are almost equal, since they are nearly an octave apart. The angles of the two semitones, however, are equal, which is inconsistent with the idea of the circular string. The names of the pitches are not given in the other editions and the manuscripts.
Descartes: diatonic scale 1
Comparison of the angles with a reference (blue), cf. related animation. The interactive example can is in the virtual lab.
Descartes: diatonic scale 2
Comparison of the angles with a reference (blue), cf. related animation. The interactive example can is in the virtual lab.
Jost Bürgi 1620
Title page of Jost Bürgi’s logarithmic tables. The red numbers form an arithmetic progression, and the black number form a geometric progression of factor 1.0001:
1.0001500 = 1.05126847
1.00011000 = 1.10516539
…
1.000123027.0022 = 9.999999997 = 10.
The diagram (or the complete tables) can be used to calculate the angles in Descartes’ diagrams. Red numbers correspond to pitch and black numbers to frequency or string length.
Marin Mersenne 1636
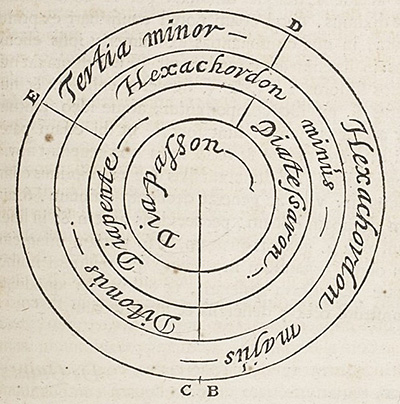
Syntonic chromatic scale of twelve notes arranged on a circle. It has four different semitones:
Chromatic semitones: 25:24 and 135:128
Diatonic semitones: 16:15 and 27:25.
The arrangement of the notes on the circle reflects a logarithmic understanding of pitch. However, the angles are neither equal to 30° (12-tet) nor do they express the four different sizes of the semitones.
The diagram is a complete analysis of the scale, where for each pair of notes (except for the semitones) both possible ratios are indicated on the connecting line.
This diagram was probably inspired by a similar diagram for the syntonic diatonic scale by Johannes Lippius (1612).
Isaac Newton 1665
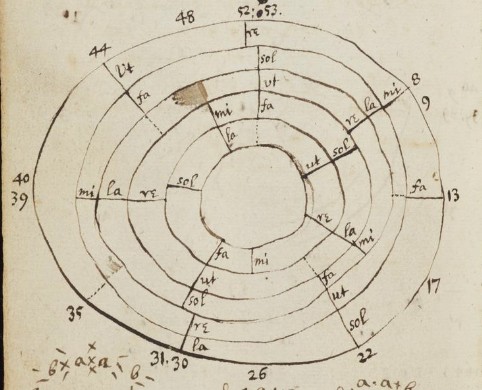
This diagram is an elaboration of Descartes’ circular hexachord diagram. Where Descartes gives three hexachords, Newton gives five full diatonic scales, ranging from two sharps (the innermost) to two flats (the outermost).
The seventh note that Newton added to Descartes’ hexachord is a diatonic semitone above the highest note la of the hexachord, and not the leading note to ut. The scales have the same interval structure T-t-S-T-t-S-T. The transpositions of the scale by fifths generate four ambiguous notes, A, B, D, E, separated by syntonic commas. The central note ut is flanked by two major tones, so that the diatonic scales contain a Pythagorean third.
The numbers assigned to the pitches are consistent with the interval sizes in 53-tet and 120-tet given on fol. 108v.
53-tet: S = 5, t = 8, T = 9 (the outer set of numbers)
120-tet: S = 1.1, t = 1.9, T = 2. (the inner set of numbers)
Isaac Newton 1665
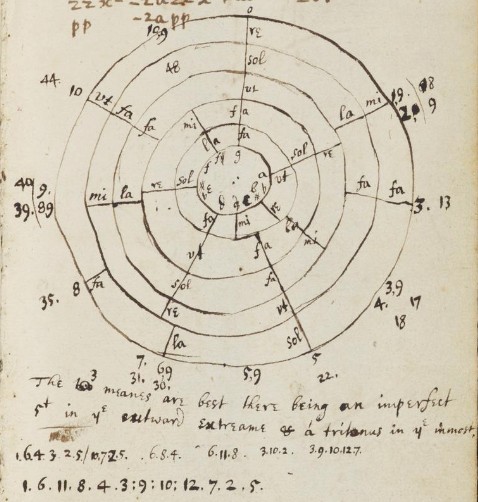
A second version of five diatonic scales in circular arrangement. This time the interval structure of the scale is t-T-S-T-t-S-T. Compared with the diagram on fol. 109r the first and second whole tone steps are exchanged, i.e., re is flattened by a syntonic comma. The seventh note of the scale is indicated with dotted lines and without solmisation. The three inner hexachords ut-re-mi-fa-sol-ut agree with Descartes’ hexachord diagram. This diatonic scale contains no Pythagorean thirds and it is the syntonic diatonic scale given by Zarlino 1562. The second degree of the corresponding major scale is a major tone above the tonic, so that Newton’s major scale is T-t-S-T-t-T-S.
The related 53-tet numbers surround the diagram. There are no 120-tet numbers and there are no note names added in this diagram.
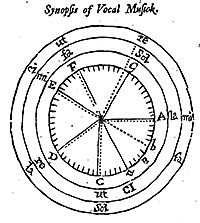
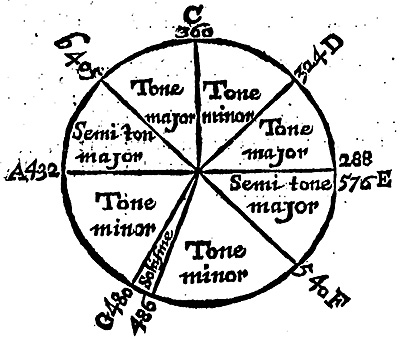
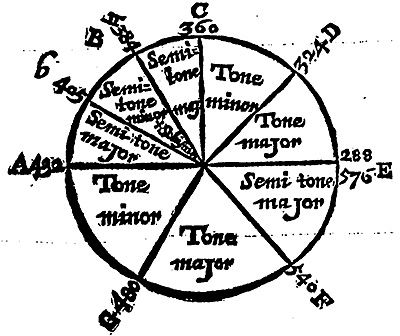
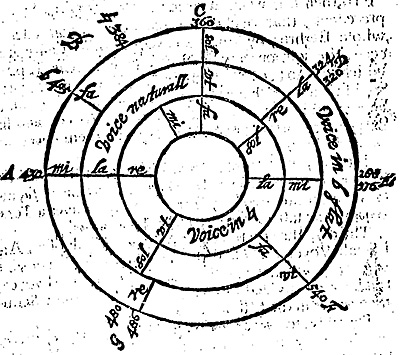
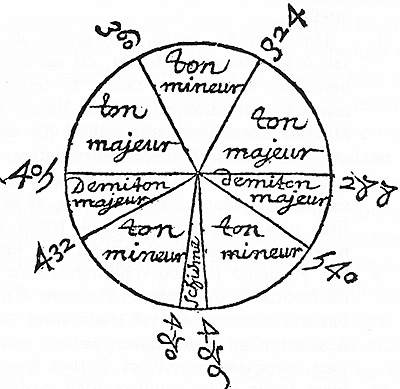
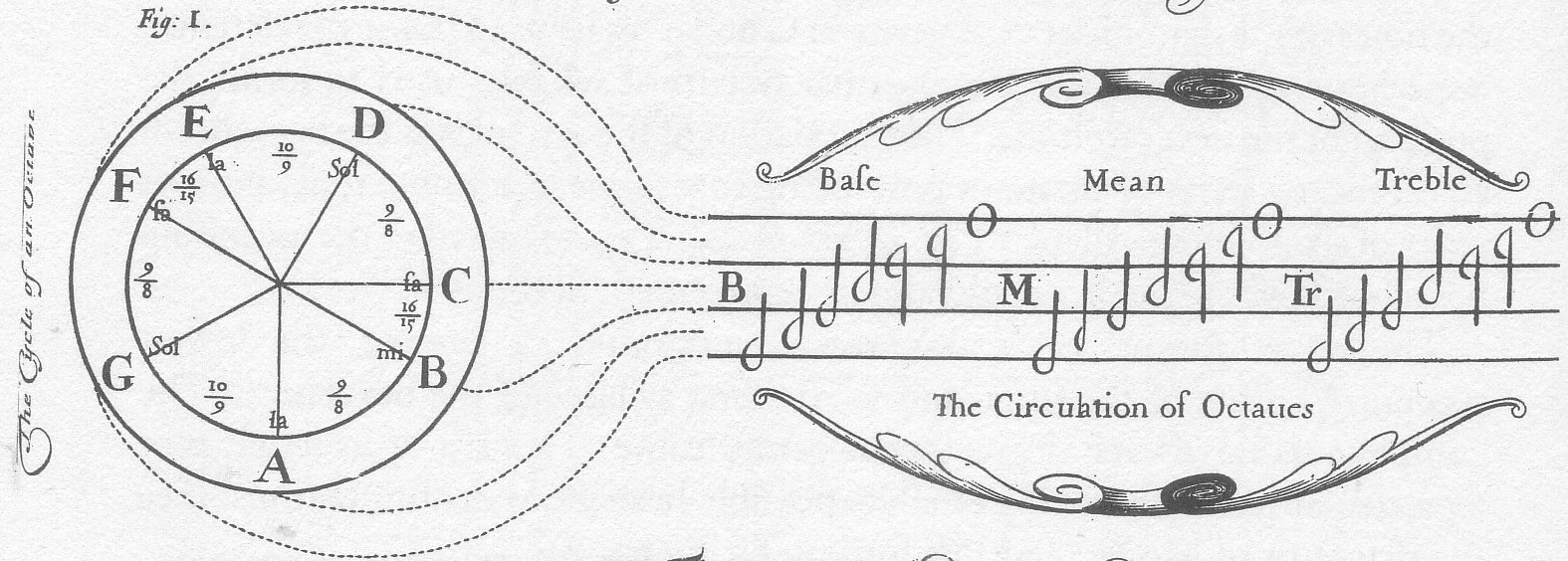
Thomas Salmon 1672
The circular pitch diagram on the left gives the syntonic diatonic scale according to Zarlino (1562) anticlockwise, with a major tone between C and D. The pitch classes are assigned to three octaves Base, Mean and Treble in the stave on the right with dotted lines. The angles in the circular diagram make no distinction between major and minor whole tones. They both measure 60° and the angles of the semitones measure 30°. These angles actually agree with the 12-tempered equal tuning, so that the scale appears to be mirror symmetric to the diameter of the circle through D.
The solmisation uses only mi, fa, sol, la, where mi-fa is a diatonic semitone (16:15), sol-la is a minor whole tone (10:9), and fa-sol as well as fa-mi are major whole tones (9:8).
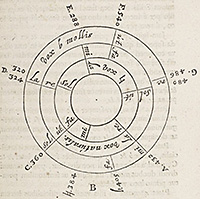
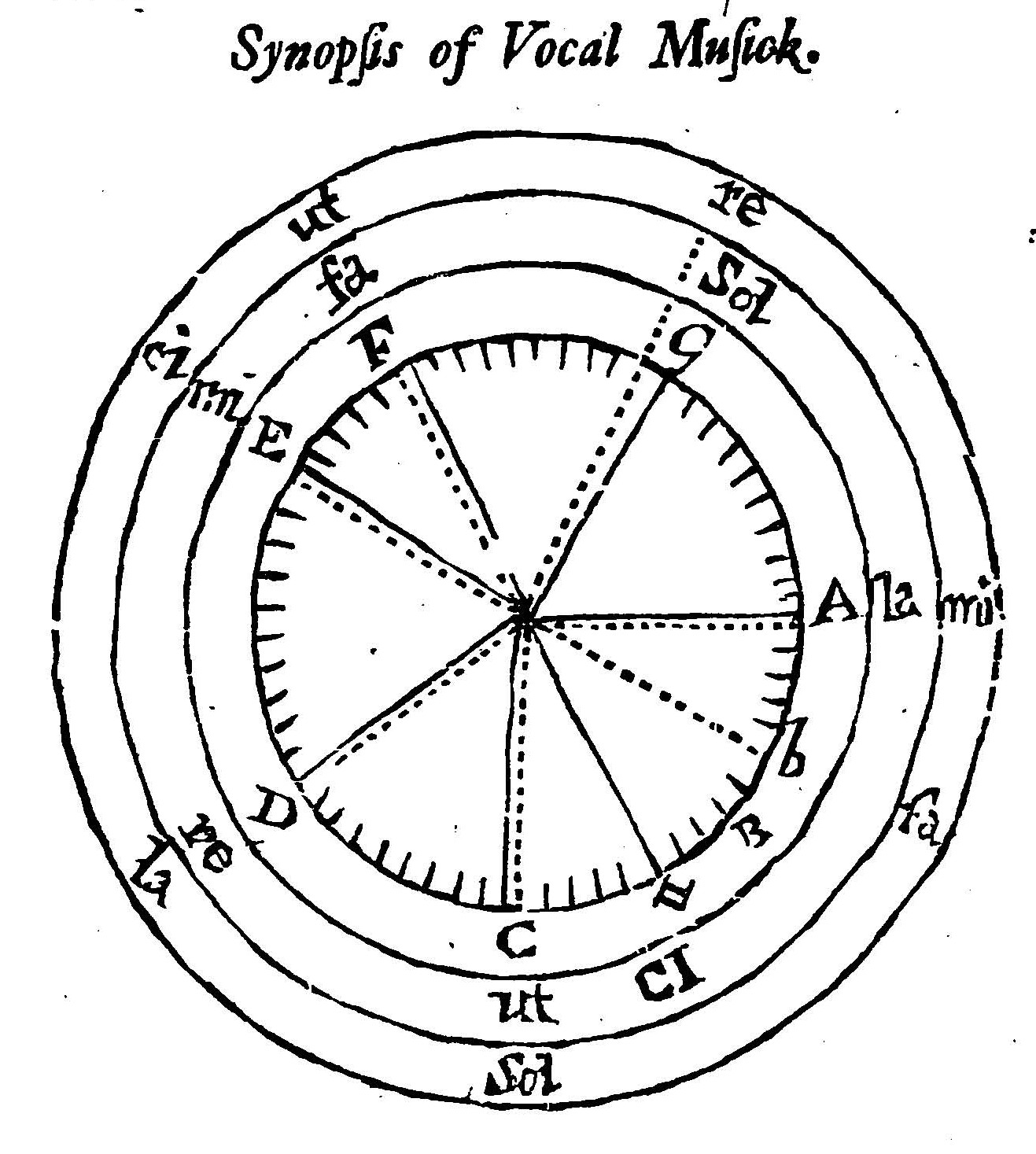
A.B. Philomus 1680
"The unidentified ‘A.B. Philomus’ included material about tuning theory in his 1680 Synopsis of Vocal Musick, including diagrams in which the octave was represented by a circle as in Descartes’ Compendium musicae. As in Descartes’ diagram different scales were shown simultaneously and compared, and the discrepancies between their versions of the ‘same’ pitches were illustrated. A notable difference is that this author also superimposes a division of the octave into many small parts: here the just intonation was intended to be executed not exactly but according to an approximation." (Benjamin Wardhaugh 2016)
In this circular pitch diagram with a scale of 59 units the major whole tone measures 10 units, the minor whole tone 9 units and the diatonic semitone 5 units. There is only one minor whole tone ut-re. There are two diatonic scales C major (solid radiuses) and F major (dashed radiuses) shown with a seven notes solmisation ut-re-mi-fa-sol-ci. They generate the same pitches as Descartes’ three hexachords, however with only one ambiguous pitch G. Major tones allow no distinction between diatonic and chromatic semitones. The minor third A-C is divided into three equal semitones.
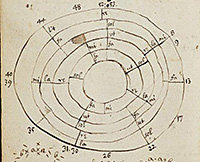
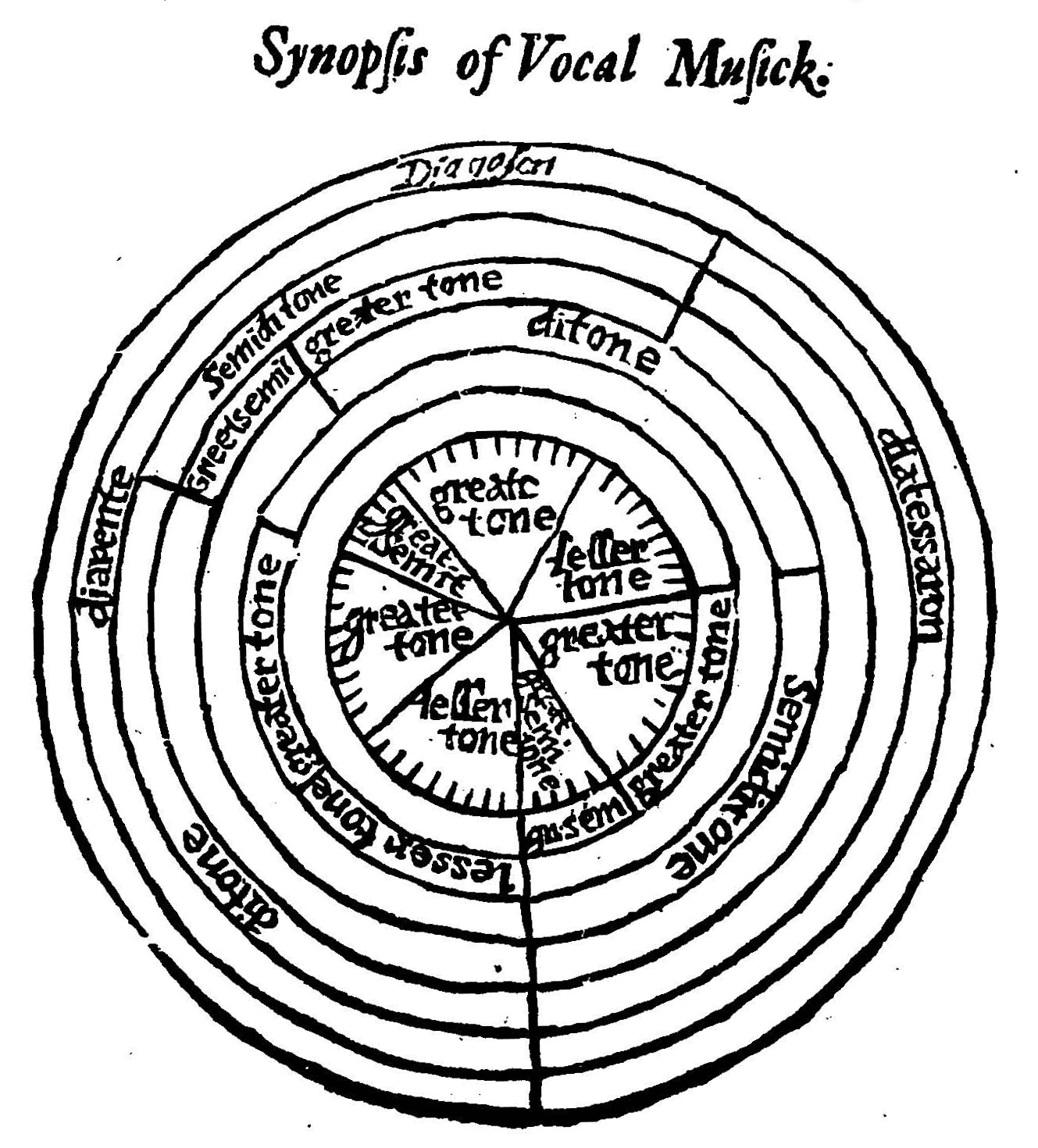
A.B. Philomus 1680
Diatonic scale in 58-tet.
This second image from the Synopsis of Vocal Musick shows the sizes of various diatonic intervals in relation to the equal division of the octave into 58 equal parts: including intervals occurring in the just intonation like the greater and lesser tones and semitones. BeW
The major tone of 10, the minor tones of 9 and the diatonic semitones of 5 units lead to a Pythagorean comma of 2 units, which does not give very accurate fifths and an inaccurate major seventh of 53 instead of 52.60 units. Differently from the division of the octave into 59 equal parts, the diatonic scale has the correct number of major and minor whole tones.
Possibly, Mersenne’s incorrect estimation of the octave as between 58 and 59 syntonic commas, was the inspiration for Philomus’ particular divisions of the octave into 58 and 59 equal parts.
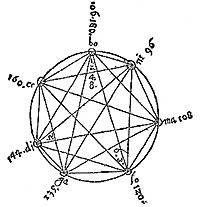
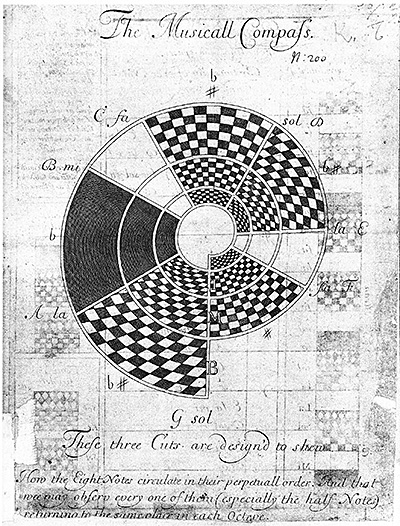
Anonymous 1684
Three octaves Base, Mean, Treble of a diatonic scale arranged on a spiral. This is the earliest known diagram which makes a precise use of the spiral as a metaphor for the octave similarity. The angles correspond to 12-tet with semitones of 30° and whole tones of 60°. The additional sharps and flats seem to refer to the same pitch. This is consistent with 12-tet or at least with a fixed chromatic scale of 12 pitches per octave.
Since the radial spacing gets narrower with increasing pitch, it is somehow related to string length, but not exactly: the radius is not halved with each full rotation.
(The Musicall Compass, British Library 557*. e.25.)
References
Anonymous (1684). The Musicall Compass. In: Herissone, Rebecca (2000). Music theory in seventeenth-century England, Oxford University Press, 2000, 85
Bürgi, Jost (1620). Aritmetische und geometrische Progress-Tabulen. Prag 1620
Cotto, Johannes (c. 1200). De musica, D-Mu 8° Cod. Ms. 375 (Cim 13), fol. 8v-27r, 35r-37v
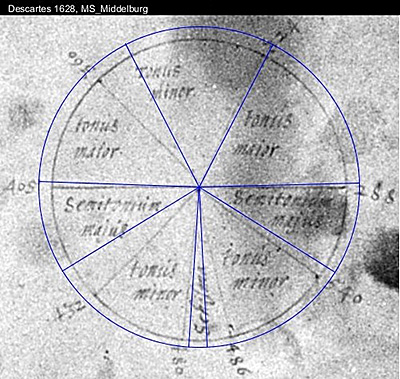
Descartes, René (1628). Ms. Middelburg, Compendium Musicae, Rene Isaco Beeckmanno, Journal de Beeckman, fol. 163r-168v
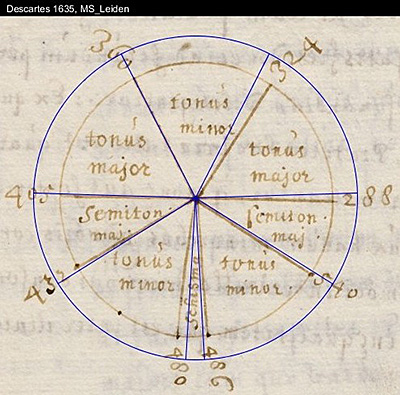
Descartes, René (1635). Compendium Musicae, Ms. Hug 29a, University Library, Leiden
Descartes, René (1640). Ms. 108, Compendium Musicae, Copy by Fr. Van Schooten Jr, University Library, Groningen
Descartes, René (1650). Renati Des-Cartes, Musicae Compendium, Trajecti ad Rhenum, Typis Gisberti à Zijll, et Theodori ab Ackersdijk, 1650
Descartes, René (1653). Renatus Des-Cartes Excellent Compendium of Musick: With Necessary and Judicious Animadversions thereupon, by a Person of Honour, London, Printed by Thomas Harper, 1653
Descartes, René (1668). Traité de la Méchanique composé par Monsieur Descartes de plus l’abregé de musique du mesme autheur mis en François avec les éclaircissemens necessaires. Traduction Nicolas Poisson, Paris, Chez Charles Angot, 1668
Durán, Domingo Marcos (1492). Lux Bella, Seville: Quatro Alemanes Compañeros, 1492
Fludd, Robert (1624). Utriusque cosmi historia, Vol I, Tract II, 1624 (first edition 1618)
Galilei, Vincenzo (1581). Dialogo di Vincentio Galilei … della musica antica, et della moderna, Firenze 1581
Lippius, Johannes (1612). Synopsis musicae novae verae atque methodicae universae, Argentorati: Paulus Ledertz, typis Carolus Kieffer, 1612
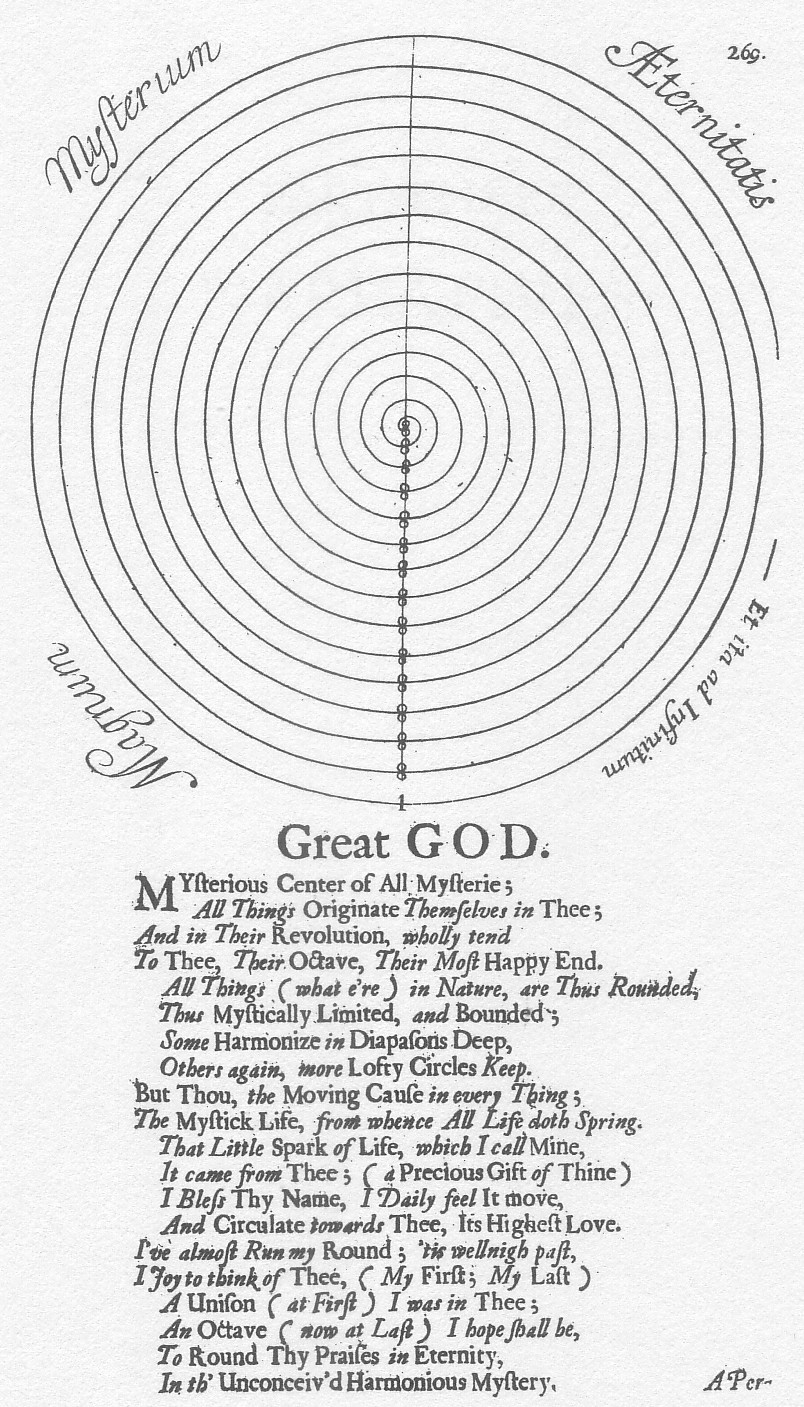
Mace, Thomas (1676). Musick’s Monument, 269. In: Gouk, Penelope (1999). Music, science, and natural magic in seventeenth-century England. Yale University Press, New Haven and London, 1999, 153
Mersenne, Marin (1636). Harmonie Universelle, contenant la Theorie et la Pratique de la Musique, Paris 1636
Newton, Isaac (1664-1655). College Notebook (MS Add.4000). Cambridge University Library
Philo-Mus, A. B. (1680). Synopsis of vocal musick, London 1680. In: Synopsis of Vocal Musick by A.B. Philo-Mus. Edited by Rebecca Herissone, Routledge 2006
Salmon, Thomas (1672). Whirligig. Vindication of an Essay (between pages 22/23). In: Gouk, Penelope (1999). Music, science, and natural magic in seventeenth-century England. Yale University Press, New Haven and London, 1999, 130
Zarlino, Gioseffo (1562). Le istitutioni harmoniche, Venetia 1562 (first edition 1558)